























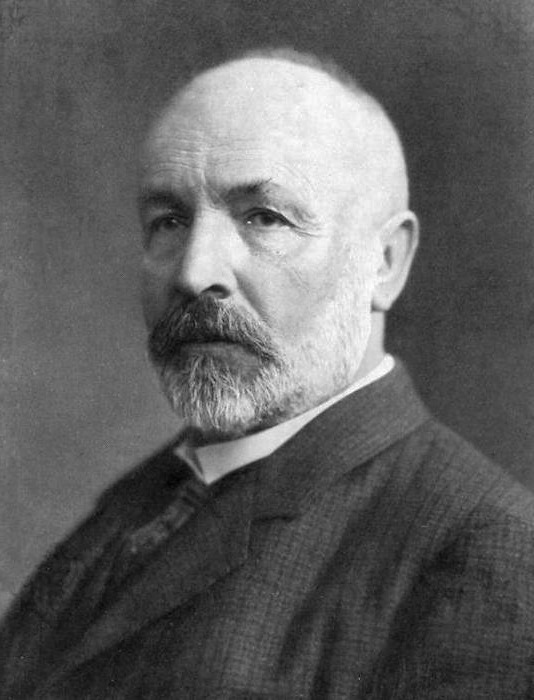






























































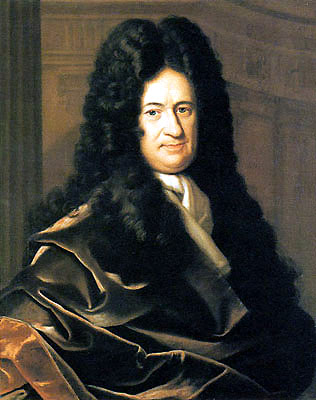




































































You probably have already heard of some of the following mathematicians, maybe all of them. You may even be familiar with their work. But can you tell in which century/ies they lived or still live, and which country they come from? The photos may give you some hints.
| Mathematician | Photo | Some key contributions |
|---|---|---|
| Abel |

|
algebraic equations; polynomials of degree 5; group theory (abstract algebra); Abelian integrals (integral calculus); elliptic integrals (integral calculus) |
| al-Khwarizmi |

|
algebraic equations; polynomials of degree 2; arithmetic; Hindu-Arabic numeral system |
| Apollonius of Perga | conic sections (geometry); book "Conics" | |
| Appell |

|
Appell series; differential equations; Appell sequence (polynomials); elliptic functions |
| Archimedes |

|
geometry; volumes of solids of revolution; trigonometry; Archimedean spiral |
| Arnold |

|
differential equations; dynamical systems; KAM theorem (integrable systems); catastrophe theory; mathematical physics |
| Artin |

|
Artinian rings (abstract algebra); algebraic number theory (abstract algebra); Galois theory; braid theory (topology) |
| Aryabhata |

|
algebraic equations; polynomials of degree 2; Diophantine equations; trigonometry |
| Banach |

|
Banach space (functional analysis); Banach algebra (functional analysis); Banach-Tarski paradox (topology) |
| Bayes |

|
Bayesian probability (probability theory) |
| Beltrami |

|
non-Euclidean geometry; Beltrami–Klein model (geometry); Singular value decomposition (matrix theory) |
| Bernoulli, Daniel |

|
probability theory |
| Bernoulli, Jacob |

|
Bernoulli numbers; constant e; Bernoulli distribution; Bernoulli differential equation |
| Bernoulli, Johann |

|
infinitesimal calculus |
| Bernoulli, Nicolaus II |

|
St. Petersburg paradox (probability theory) |
| Bessel |

|
Bessel functions (special functions) |
| Bézout |

|
algebraic equations; Bézout's identity (number theory) |
| Bhaskara II | algebraic equations; polynomials of degree 2; Diophantine equations; Pell's equation | |
| Bienaymé |

|
Bienaymé-Chebyshev inequality (probability theory); Bienaymé formula (statistics) |
| Binet |

|
matrix multiplication; Cauchy-Binet formula (matrix theory); matrix algebra; Binet-Cauchy identity; Binet's Fibonacci number formula (number theory); Binet equation (differential equation) |
| Bolyai |

|
non-Euclidian geometry; complex analysis |
| Bolzano |

|
foundations of mathematics; limit of a function; Bolzano-Weierstrass theorem |
| Boole |

|
Boolean algebra; mathematical logic |
| Borel |

|
Borel set (topology); measure theory; probability theory |
| Brahmagupta | algebraic equations; polynomials of degree 2; Diophantine equations; Pell's equation; arithmetic; use of 0 | |
| Brioschi |

|
elliptic functions; polynomials of degree 5; polynomials of degree 6 |
| Brouwer |

|
topology; Brouwer's fixed-point theorem (algebraic topology); simplicial approximation theorem (algebraic topology); invariance of domain (topology) |
| Cantor |
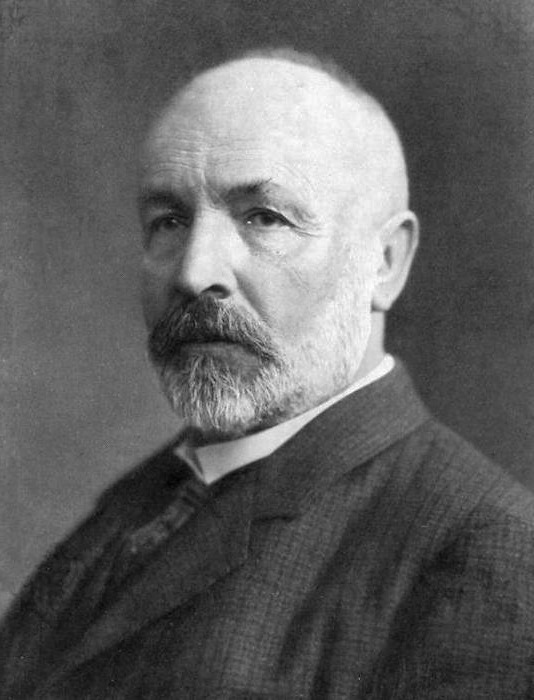
|
foundations of mathematics; axiomatization; set theory; transfinite numbers; cardinal numbers; ordinal numbers; transcendental numbers |
| Cardano |

|
algebraic equations; polynomials of degree 3 |
| Cartan |

|
Cartan matrix; group theory (abstract algebra); Cartan decomposition (abstract algebra); Cartan's theorem (abstract algebra) |
| Cauchy |

|
foundations of mathematics; series; complex analysis; infinitesimal calculus; limit of a function; Cauchy sequence; continuity; Cauchy-Schwarz inequality; group theory (abstract algebra) |
| Cayley |

|
group theory (abstract algebra); Cayley's theorem (group theory); Cayley-Hamilton theorem (matrix theory); Cayley graph (graph theory); Cayley's formula (graph theory) |
| Cesàro |

|
differential geometry; Cesàro mean (divergent series) |
| Chasles |

|
Chasles's relation (geometry); cross-ratio (geometry); coined the term "homothety" |
| Chebyshev |

|
Chebyshev polynomials; orthogonal polynomials; Bienaymé-Chebyshev inequality (probability theory); Chebyshev function (number theory); Chebyshev's bias (number theory) |
| Clairaut |

|
Clairaut's equation (differential equation); Clairaut's relation (differential geometry) |
| Cramer |

|
Cramer's rule (matrix theory) |
| D'Alembert |

|
fundamental theorem of algebra; d'Alembertian |
| Darboux |

|
Darboux sums (integral calculus); Darboux integral (integral calculus); Darboux's formula (series and integral calculus); Euler-Poisson-Darboux equation (differential equations); differential geometry of surfaces |
| Dedekind |

|
foundations of mathematics; set theory; ring theory (abstract algebra); number theory |
| Del Ferro | algebraic equations; polynomials of degree 3 | |
| De Moivre |

|
de Moivre's formula (trigonometry); Binet's formula (number theory); probability theory |
| De Morgan |

|
De Morgan's laws; mathematical logic; mathematical induction |
| Descartes |

|
Cartesian geometry; convention of using x, y, z etc. for unknowns in equations and a, b, c, etc. for knowns |
| Dieudonné |

|
Dieudonné module (abstract algebra); Dieudonné ring (abstract algebra); books |
| Diophantus | algebraic equations; polynomials of degree 2; Diophantine equations | |
| Dirichlet |

|
number theory; Dirichlet L-functions; Fourier series; continuity; Dirichlet integral (integral calculus) |
| Dudeney |

|
recreational mathematics |
| Eisenstein |

|
Eisenstein criterion (polynomials); quadratic reciprocity law; number theory |
| Eratosthenes |

|
sieve of Eratosthenes (number theory) |
| Erdélyi | special functions; orthogonal polynomials; hypergeometric functions | |
| Erdös |

|
graph theory; number theory; Prime Number Theorem; probability theory |
| Euclid |

|
arithmetic; number theory; trigonometry; Euclidian geometry |
| Eudoxus of Cnidus | geometry; method of exhaustion (integral calculus) | |
| Euler |

|
infinitesimal calculus; Seven Bridges of Königsberg (graph theory); number theory; Euler's totient function; power series; Euler-Maclaurin formula (series and integral calculus); transcendental numbers; concept of mathematical function |
| Faltings |

|
number theory; Mordell conjecture |
| Faulhaber |

|
Faulhaber's formula (sums of powers) |
| Fejér | harmonic analysis; Fejér kernel (Fourier series); Fejér's theorem | |
| Fermat |

|
Diophantine equations; Pell's equation; Fermat's little theorem (number theory); Fermat's theorem on sums of two squares (number theory); Fermat numbers (number theory); Fermat's Last Theorem (number theory) |
| Ferrari | algebraic equations; polynomials of degree 4 | |
| Fibonacci |

|
Fibonacci numbers; Hindu-Arabic numeral system |
| Fourier |

|
Fourier series; Fourier transform |
| Fraenkel |

|
mathematical logic; foundations of mathematics; axiomatization; Zermelo-Fraenkel axioms (set theory) |
| Freedman |

|
geometric topology; Poincaré conjecture for n=4 |
| Frobenius |

|
elliptic functions; differential equations; Frobenius algebra (abstract algebra); Perron–Frobenius theorem (matrix theory) |
| Galois |

|
algebraic equations; polynomials of any degree; Galois theory |
| Gardner |

|
recreational mathematics |
| Gauss |

|
fundamental theorem of algebra; number theory; quadratic forms; modular arithmetic; convention of using ≡ for congruence; geometry; Gaussian curvature (differential geometry); differential geometry of surfaces; Gauss-Jordan elimination (matrix theory) |
| Gelfand |

|
group theory; representation theory; functional analysis |
| Germain |

|
number theory; Fermat's last theorem (number theory); differential equations |
| Gödel |

|
mathematical logic; foundations of mathematics; Gödel's incompleteness theorems |
| Goldbach | Goldbach's conjecture; Fermat numbers (number theory) | |
| Green | Green's theorem (integral calculus); Green's identities (integral calculus) | |
| Grothendieck |

|
algebraic geometry; algebraic number theory (abstract algebra) |
| Hadamard |

|
prime number theorem (number theory); complex analysis; differential geometry; calculus of variations; differential equations |
| Hamilton, Richard |

|
differential geometry; Ricci flow (differential geometry) |
| Hamilton, William |

|
Hamiltonian; quaternions; hamiltonian paths (graph theory); Hamilton-Jacobi equation (differential equation) |
| Hardy |

|
number theory; analysis; Waring's problem; Hardy–Littlewood conjectures |
| Hausdorff |

|
Hausdorff space (topology); Hausdorff maximal principle (set theory); Hausdorff measure (measure theory) |
| Hermite |

|
Hermitian matrices; Hermite normal form; Hermite polynomials; Hermitian forms; transcendental numbers |
| Heron of Alexandria |

|
square roots; algebraic equations; polynomials of degree 2; Heron's formula |
| Hilbert |

|
foundations of mathematics; axiomatization; Hilbert space; functional analysis; Hilbert's 23 problems |
| Hipparchus |

|
trigonometry |
| Hölder |

|
abstract algebra; classification of simple groups (group theory); Hölder's inequality (analysis); Hölder condition (analysis); Hölder's theorem (gamma function) |
| Ito | probability theory; stochastic differential equations; Ito's lemma | |
| Iwasawa | Iwasawa decomposition (abstract algebra); Iwasawa algebra (abstract algebra); Iwasawa theory (abstract algebra) | |
| Jacobi |

|
elliptic functions; Hamilton-Jacobi equation (differential equation); Jacobian matrix; Jacobian (determinant); Jacobi symbol |
| Jordan, Camille |

|
group theory (abstract algebra); Jordan matrix; Jordan's totient function; Jordan curve theorem (topology) |
| Jordan, Wilhelm |

|
Gauss-Jordan elimination (matrix theory) |
| Klein |

|
non-Euclidean geometry; Klein bottle (geometry); Erlangen program (geometry); Beltrami–Klein model (geometry); group theory (abstract algebra) |
| Kolmogorov |

|
probability theory; differential equations; KAM theorem (integrable systems); stochastic processes |
| Kovalevskaya |

|
Cauchy–Kowalevski theorem (differential equations); Abelian integrals (integral calculus) |
| Kronecker |

|
Kronecker δ; Kronecker product (matrix theory); Kronecker symbol (number theory); algebraic number theory (abstract algebra) |
| L'Hôpital |

|
L'Hôpital's rule (infinitesimal calculus) |
| Lagrange |

|
Lagrange's four-square theorem (number theory); calculus of variations; Euler-Lagrange equation (differential equation); Lagrange multipliers (mathematical optimization); Lagrangian |
| Lang |

|
abstract algebra; Diophantine geometry; modular forms; books |
| Langlands | abstract algebra; Langlands program (algebra and analysis) | |
| Laplace |

|
Laplacian; Laplace transform; Bayesian probability (probability theory) |
| Laurent | Laurent series (complex analysis); Laurent polynomial | |
| Lebesgue |

|
Lebesgue integration (integral calculus); measure theory |
| Legendre |

|
least squares method; Legendre polynomials; quadratic reciprocity law; elliptic functions; Legendre symbol |
| Lehmer |

|
number theory; primality tests; Lucas-Lehmer test; Mersenne primes |
| Leibniz |
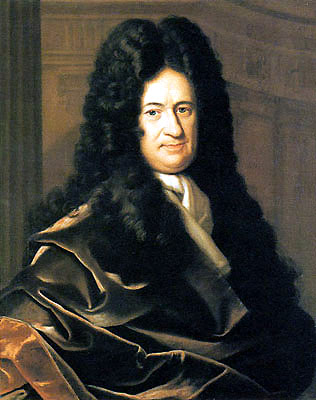
|
infinitesimal calculus; convention of using d for differentials (infinitesimal calculus); convention of using an elongated S for integrals (integral calculus) |
| Levi-Civita |

|
tensor calculus; Hamilton–Jacobi equation |
| Lie |

|
Lie groups (abstract algebra); group theory (abstract algebra) |
| Lions |

|
nonlinear partial differential equations |
| Liouville |

|
number theory; complex analysis; Liouville's theorem; transcendental numbers; Liouville numbers; Sturm-Liouville theory |
| Lipschitz |

|
Lipschitz continuity condition; Dini-Lipschitz criterion |
| Littlewood | number theory; analysis; Diophantine approximation; Waring's problem; Hardy–Littlewood conjectures | |
| Lobachevsky |

|
hyperbolic geometry (non-Euclidean geometry) |
| Lucas |

|
Diophantine equations; number theory; primality tests; Lucas sequences; Lucas numbers; Lucas-Lehmer test; Mersenne primes |
| Lyapunov |

|
differential equations; Lyapunov exponent (chaos theory); central limit theorem (probability theory) |
| Maclaurin |

|
Maclaurin series; Euler-Maclaurin formula (series and integral calculus) |
| Manin |

|
algebraic geometry; arithmetic topology; Diophantine geometry; Gauss-Manin connection |
| Markov |

|
Markov chains; Markov processes; stochastic processes |
| Matiyasevich |

|
Hilbert's tenth problem; Diophantine equations |
| Mazur |

|
geometric topology; arithmetic topology; Diophantine geometry |
| Mersenne |

|
Mersenne primes |
| Minkowski |

|
Minkowski inequality; number theory |
| Mittag-Leffler |

|
Mittag-Leffler function (special functions); Mittag-Leffler star (complex analysis); Mittag-Leffler's theorem (complex analysis); Mittag-Leffler summation (formal power series) |
| Mordell |

|
number theory; Diophantine equations; Mordell curve; modular forms; Mordell-Weil theorem; Mordell conjecture |
| Napier |

|
logarithm; decimal point |
| Newton |

|
dynamical systems; infinitesimal calculus; binomial theorem |
| Noether |

|
abstract algebra; Noetherian ring (abstract algebra) |
| Ostrogradsky |

|
divergence theorem; calculus of variations |
| Painlevé |

|
differential equations |
| Pascal |

|
probability theory; Pascal's triangle |
| Peano |

|
mathematical logic; foundations of mathematics; set theory; Peano axioms (axiomatization); Peano existence theorem (differential equations) |
| Perelman |

|
geometric topology; Poincaré conjecture for n=3; Thurston's geometrization conjecture (geometric topology) |
| Perron |

|
Perron method (differential equations); Perron–Frobenius theorem (matrix theory); Perron's formula (number theory) |
| Poincaré |

|
dynamical systems; Poincaré map (chaos theory); topology; Poincaré conjecture; fundamental group (algebraic topology); Fuchsian groups; Kleinian groups; differential equations |
| Poisson |

|
differential equations; Poisson's equation; probability theory; Poisson distribution |
| Pólya |

|
heuristics; combinatorics; number theory; probability theory |
| Pythagoras |

|
arithmetic; Pythagorean theorem |
| Ramanujan |

|
number theory; series; continued fractions; Ramanujan-Petersson conjecture |
| Ribet |

|
Fermat's last theorem (number theory); modular forms; Taniyama-Shimura conjecture (topology and number theory) |
| Riccati |

|
Riccati equation (differential equation) |
| Ricci-Curbastro |

|
tensor calculus; Ricci flow (differential geometry); Ricci curvature (differential geometry) |
| Riemann |

|
Riemannian geometry (non-Euclidean geometry); Riemann zeta function; Riemann hypothesis; Riemann integral (integral calculus); real analysis; differential geometry of surfaces; |
| Riesz | divergent series; partial differential equations | |
| Rolle | Rolle's theorem | |
| Russell |

|
mathematical logic; foundations of mathematics; Russell's paradox |
| Sarrus | rule of Sarrus (determinants); Sarrus numbers (number theory) | |
| Schwartz |

|
theory of distributions |
| Serre |

|
algebraic geometry; algebraic number theory (abstract algebra) |
| Shimura |

|
Fermat's last theorem (number theory); Taniyama-Shimura conjecture (topology and number theory) |
| Simpson | Simpson's rule (integral calculus) | |
| Smale |

|
geometric topology; h-cobordism; Poincaré conjecture for n≥5 |
| Sobolev |

|
theory of distributions; Sobolev space (analysis) |
| Stallings |

|
geometric group theory; Stallings theorem about ends of groups (group theory); geometric topology; Poincaré conjecture for n=6 |
| Stewart |

|
recreational mathematics |
| Stieltjes |

|
Riemann-Stieltjes integral (integral calculus); continued fractions; orthogonal polynomials |
| Stirling | Stirling numbers; Stirling permutations; Stirling's approximation (factorials) | |
| Stokes |

|
Stokes's theorem (differential geometry); Stokes line (complex analysis) |
| Sturm |

|
Sturm's theorem (polynomials); Sturm-Liouville equation (differential equation); Sturm-Liouville theory (S-L theory); Sturm series (polynomials) |
| Sun Zi | algebraic equations; Diophantine equations; square roots; Chinese remainder theorem (number theory); books | |
| Sylvester |

|
Sylvester's determinant theorem (matrix theory); Sylvester's formula (matrix theory); Sylvester equation (matrix theory); coined the terms "graph", "discriminant", and "totient" |
| Szegö | orthogonal polynomials; Toeplitz matrices | |
| Taniyama |

|
Fermat's last theorem (number theory); Taniyama-Shimura conjecture (topology and number theory) |
| Tao |

|
Green-Tao theorem (number theory); circular law (probability theory); Hardy-Littlewood prime tuples conjecture; prime gaps (number theory) |
| Tartaglia |

|
algebraic equations; polynomials of degree 3 |
| Taylor, Brook |

|
Taylor series; Taylor's theorem |
| Taylor, Richard |

|
Fermat's last theorem (number theory); Taniyama-Shimura conjecture (topology and number theory); Langlands program (algebra and analysis) |
| Thales |

|
geometry |
| Thom |

|
topology; catastrophe theory; singularity theory |
| Thue |

|
Diophantine equations; Diophantine approximations; Thue equation; Thue-Siegel-Roth theorem |
| Thurston |

|
manifolds (topology); foliation theory (topology); Thurston's geometrization conjecture (geometric topology) |
| Turán |

|
number theory; graph theory |
| Vandermonde | Vandermonde matrix; Vandermonde determinant; Vandermonde's identity | |
| Van der Waerden |

|
abstract algebra |
| Viète |

|
convention of using letters for unknowns in equations |
| Vinogradov | analytic number theory | |
| Von Neumann |

|
foundations of mathematics; measure theory; ergodic theory |
| Wallis |

|
approximation of π; convention of using symbol ∞ for infinity; infinitesimal calculus |
| Weierstrass |

|
foundations of mathematics; axiomatization; limit of a function; analysis; Weierstrass factorization theorem (complex analysis); Bolzano-Weierstrass theorem; elliptic functions; calculus of variations |
| Weil |

|
number theory; algebraic geometry; Mordell-Weil theorem; Weil conjectures |
| Weyl |

|
Riemann surfaces (topology); compact groups (abstract algebra); Weyl groups (abstract algebra); Lie algebras (abstract algebra); Weyl law (eigenvalues); Weyl's criterion (Diophantine equations) |
| Wiles |

|
Fermat's last theorem (number theory); Taniyama-Shimura conjecture (topology and number theory) |
| Yoccoz |

|
dynamical systems |
| Zeeman |

|
geometric topology; Poincaré conjecture for n=5; catastrophe theory; singularity theory |
| Zermelo |

|
mathematical logic; foundations of mathematics; axiomatization; Zermelo-Fraenkel axioms (set theory) |
Answer: